Zeno’s Paradoxes

– Alihyder Mulji

Paradoxes since time immemorial have puzzled and fascinated humans. A paradox is a statement which is seemingly absurd but when investigated, may prove to be true. In this blog, we will read some interesting paradoxes proposed by Zeno. Zeno of Elea was a Greek Philosopher who is best known for his paradoxes. Although most of these paradoxes have been ‘solved’, it is fun to revisit theories that kept philosophers thinking for hours on end.

The Dichotomy Paradox
Let’s look at the story of the tortoise and the hare to understand this paradox.
Let’s say the tortoise in our story didn’t really win, but got a head start of 1m because the hare was sleeping.

Since the hare runs faster than the tortoise, it covers more distance in the same time. But by the time the hare covers certain amount of distance, the tortoise moves a small distance further in the same time.
After a finite amount of time the distance between the two is halved (1/2).

A few moments later, it has reduced to 1/4 (0.25m) , 1/8 (0.125m) , 1/16 (0.0625m) and so on-

Since there are infinite numbers in mathematics, the distance continues to reduce (0.0625, 0.03125, 0.015625, 0.0078125…) further and further and the time taken to cover the distance keeps adding up. Since the distance can continue to reduce an infinite number of times (until we reach 1/n where n tends to infinity) the time taken will be obtained by adding these infinite divisions. Therefore the time taken to catch up with the tortoise should be infinite.
Does this mean the hare never really catches up with the tortoise? Is this the real reason he lost the race?
Logically, you would reply no because in the real world, the hare will eventually catch up and even overtake the tortoise. But where is the flaw in the logic? How can you prove mathematically that the hare does catch up? This is a paradox because the fact that the hare can catch up with the tortoise seems unlikely at first, it can be solved mathematically.

Solution
Lets look at a solution for this paradox.
If were to make an equation for the distance (S) traveled by the hare and perform some basic operations it would look like this-

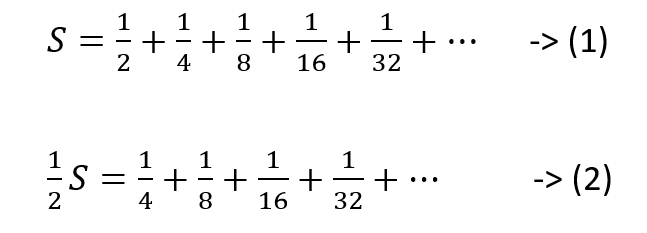
The first time the hare travels half a meter, then a quarter, then an eighth and so on.
This is the (1) equation.
If we divide the first equation into half we get the (2) equation.
Now, if you subtract (2) from (1), we get-

Because numbers from ¼ to 1/n (where n tends to infinity) get cancelled out.
Eventually giving us-

We can therefore, mathematically conclude that the hare does travel a distance of 1 meter to bridge the gap between him and the tortoise. This is the use of limits to solve the dichotomy paradox. Adding up extremely small numbers is also covered under integration and therefore the paradox can also be solved using that.

(This Paradox is often called the Paradox of Achilles and The Tortoise)

A similar example would be cutting up a square in half repeatedly.

The Paradox of No motion
Imagine a marksman trying to hit his target with an arrow. The arrow first needs to be released from the bow and then it will be set in motion to reach its target. Now, lets imagine the arrow at 3 different points.

Let’s consider each of these points as a single instant. At a single instant, there is no motion and the arrow takes up the space which is equal to its size. This means that the arrow is at rest. If there is no motion at one instant, there is no motion at all because time is made up of several instances, and therefore motion is an illusion.

We can clearly see that the arrow moves to its target, but where is the flaw in the logic above?
This paradox is solved with the concept of instantaneous speed, which means speed of an object at a given moment of time.

Isn’t it fascinating to think how such simple situations have baffled philosophers for hundreds of years? What do you think about these paradoxes? Let us know in the comments below and stay tuned for more such articles from MSCNM!

Sources:

https://www.wikipedia.org/

https://www.ted.com/

https://www.youtube.com/user/numberphile